A short introduction to Computational Aeroacoustics (CAA)
- advantages of zonal approaches

Requirements for sound simulation

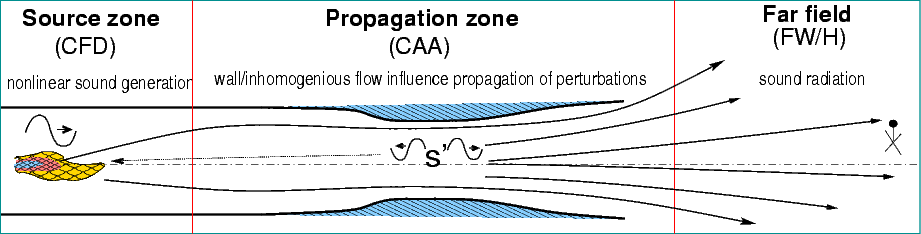
In order to provide a reliable simulation of the far-field propagation and radiation of an internal sound source through an engine inlet or outlet, the following characteristics are desirable:

• Good far-field prediction
• Reasonable computing time
• Reproduction of sound characteristics
• Conservation of the dispersion relation
• Propagation without dissipation

Domain decomposition

The correct reproduction of sound characteristics unfortunately necessitates expensive requirements on the numerical simulation. In an attempt to reduce this computational burden, a decomposition of the computational domain with respect to the differing modelling requirements and numerical constraints is carried out. Even though the problem of sound propagation to the far-field is usually large, this approach allows an accurate simulation with an optimized numerical approach for each of the different regions.

Sound source

The unsteady flow responsible for various noise sources (e.g. around the fan blades, rotor-stator interaction, buzz-saw noise, combustion chambers, turbines...) is simulated using the unsteady Navier-Stokes equations together with a range of turbulence-handling techniques (link to turb-mod?). Such high-resolution CFD simulations are very expensive, however only necessary for the relatively confined source region.

Propagation zone

The data from this source simulation is input to the sound propagation calculation. The steady mean flow and its interaction with soft or hard walls influences the sound propagation. These effects can be modelled in a linearised manner using disturbance equations (LEE, APE (Ewert & Schröder, 2003)). The acoustic scales of interest are usually much larger, due to their propagation at the speed of sound relative to the medium. Neglecting the influence of slowly-propagating hydrodynamic disturbances, this approach allows the simulation of sound propagation with a dramatically reduced number of grid points. This makes the simulation of sound propagation with an optimized CAA method much more efficient (Hu et al., 1996), and therefore less expensive then the CFD methods used in the source region.

Far-field

The sound field in the intake and in the vicinity of the engine is not sufficient for most applications, where the far-field noise levels are of importance. Under the assumption of a uniform mean flow and neglecting wall effects on the sound propagation, the sound radiation to the far-field is modelled by the aeroacoustic analogy of Ffowcs Williams & Hawkings. The solution by such an integral method is less expensive than CAA and produces no amplitude decay. The basis for correct prediction at a given observer position is nonetheless a correct sound field in the intake and in the near field of the engine. Because of the decomposition of the domain, the total error is an accumulation of the errors produced by each of the methods, however the capabilities and advantages of each methods have been exploited to full capacity.